Publication Date: May 8, 2024
Authors: Kouhei Nakaji, Mohsen Bagherimehrab, and Alán Aspuru-Guzik
Abstract:
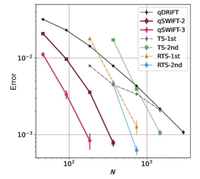
Hamiltonian simulation is known to be one of the fundamental building blocks of a variety of quantum algorithms such as its most immediate application, that of simulating many-body systems to extract their physical properties. In this work, we present qSWIFT, a high-order randomized algorithm for Hamiltonian simulation. In qSWIFT, the required number of gates for a given precision is independent of the number of terms in the Hamiltonian, while the systematic error is exponentially reduced with regard to the order parameter. In this respect, our qSWIFT is a higher-order counterpart of the previously proposed quantum stochastic drift protocol (qDRIFT), the number of gates in which scales linearly with the inverse of the precision required. We construct the qSWIFT channel and establish a rigorous bound for the systematic error quantified by the diamond norm. qSWIFT provides an algorithm to estimate given physical quantities by using a system with one ancilla qubit, which is as simple as other product-formula-based approaches such as regular Trotter-Suzuki decompositions and qDRIFT. Our numerical experiment reveals that the required number of gates in qSWIFT is significantly reduced compared to qDRIFT. In particular, the advantage is significant for problems where high precision is required; e.g., to achieve a systematic relative propagation error of 10−6, the required number of gates in third-order qSWIFT is 1000 times smaller than that of qDRIFT.
Related links: